What is a Ray in Geometry?

Definition of a Ray in Geometry

Mathematical Definition

Geometry defines a ray as part of a line which extends in one direction infinitely from an endpoint and never ends; unlike lines which extend infinitely in both directions or line segments with fixed endpoints, its length cannot be accurately determined for this geometry feature which provides directionality with endless extension in one specific direction.

Imagine a straight road that begins from one marked point (its endpoint), leading indefinitely in one direction; such a roadway could be described as an infinite ray.

To better comprehend rays, imagine them as "one-way streets" of geometry; each represents an infinitely moving path forward from where it began. Furthermore, their shape corresponds with time moving ahead while the past remains static while future possibilities abound - an analogy which provides clarity of understanding rays.

Ray Notation in Geometry

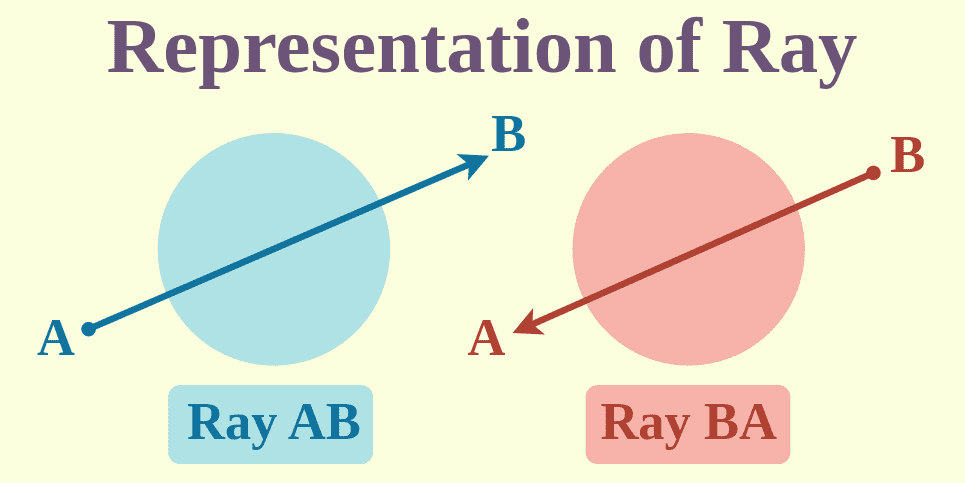
Rays are represented using a specific notation, with the endpoint listed first. For example, if a ray starts at point A and passes through point B in an infinite direction, it is denoted as AB →. Note the arrow pointing to the right, which signifies the infinite extension in that direction. Importantly, the order matters—AB → is not the same as BA → because the starting point (A) must always be listed first to indicate the directionality of the ray.

To solidify this understanding, imagine drawing a line on paper. Fix point A as the origin and draw the line toward point B, adding an arrow at the end. This visualization represents the ray AB →, where the direction is clearly from A to B.

Real-Life Examples of Rays

Practical Observation of Rays

Rays aren't simply theoretical concepts in geometry; they appear everywhere, from natural environments to man-made buildings around us, and help us better comprehend their core principle: Rays help us conceptualize them intuitively:

Sunlight

The sun offers us an ideal real-life example of a ray. As its endpoint remains fixed, emitting continuous light rays which travel infinitely outward in straight lines from its centre point - this expansiveness mirroring geometric rays' endless properties. On sunny days, its interaction with objects produces shadows which help us visualize how rays extend one direction.

Laser Pointers

Laser pointers offer an apt illustration of ray action. Once activated, their laser beam creates an intensely focused stream of light emanating from one point (typically its light-emitting source) before radiating indefinitely in all directions - the perfect illustration of focused travel along an invisible ray's trajectory.

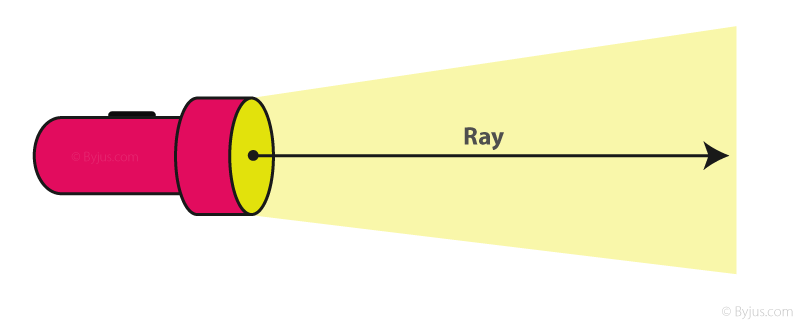
Flashlights

As soon as a flashlight is activated in a dark room, its beam of light expands out from its bulb in an orderly and direct fashion, acting like an exact representation of a geometric ray originating at one single point and projecting light along a path with no breaks along its trajectory. Even as its directionality dissipates over long distances.

Applications in Daily Life

Beyond direct and obvious physical examples, rays exhibit subtle yet critical applications in our daily lives. These examples highlight how we continuously interact with ray-like structures, often without noticing:

Road Navigation

Modern navigation devices, including GPS systems, use ray-like pathways to help us plan and follow routes. A route displayed by these systems begins from your current location (which serves as the fixed starting point), projecting outward toward your destination in one specific direction - similar to how rays behave geometrically - until it eventually reaches it's endpoint - further cementing directionality's importance while traversing roads as it ensures alignment between representation of infinite extension.

Railway Tracks

A pair of straight railway tracks extending toward the horizon evokes the image of a ray. From the observer's perspective, the tracks visually appear to start from a fixed point and stretch into infinity in a single direction—even though they may eventually curve or stop.

Lighthouse Beams

Lighthouses provide another subtle yet striking example of rays in action. A lighthouse beam starts from the central source of light and travels outward over the vast ocean, guiding sailors in a clear and specific direction. The focused beam can be seen as a representation of directional rays, aiding navigation much like rays in geometry guide our understanding of direction.

Water Hoses

Imagine water coming out of a hose nozzle as a stream that extends in one defined direction from its point of release, eventually dissipating but beginning as an evident path akin to geometric rays with fixed points of origin.

Surveillance Cameras

Many modern security systems use infrared beams for monitoring. These beams extend in specific directions from the camera's source, creating invisible "rays" of detection. The properties of these beams—straight paths radiating from a specific endpoint—are similar to rays in geometry.

At first glance, rays appear everywhere: from tangible forms like light to more abstract representations like navigation systems. Rays as foundational geometric constructs not only help us comprehend mathematical principles better but define and improve many systems we encounter daily in life.

Properties of Rays

Fundamental Characteristics

Rays possess distinct features that differentiate them from other basic geometric elements like lines and line segments. Below are their key properties:

Single Endpoint

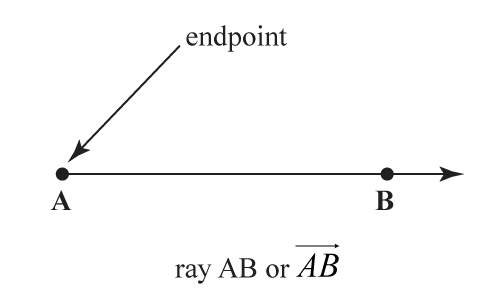
A ray always begins at one fixed point, referred to as the endpoint. This starting point acts as the origin of the ray and is a defining feature that sets it apart from a line, which has no endpoints. For instance, in the ray AB →, point A is the fixed starting point.

Infinite Extension

An infinite ray is different than its linear equivalent in that it extends indefinitely in one direction without boundaries; once leaving an endpoint, it continues with no boundaries in that chosen direction, usually represented as an arrow on graph paper.

Unmeasurable Length

As the length of a ray extends indefinitely in one direction, its measurement cannot be accurately quantified or assessed compared with line segments, which have fixed endpoints that allow measurements such as these.

Directional Nature

A ray has a clear and specific direction determined by its starting endpoint and the path it follows. This directional feature is crucial in determining angles, graphing inequalities, and other applications in geometry. For instance, the direction of AB → is from A toward B, and it cannot reverse or deviate.

Equality of Rays

Two rays are considered equal if and only if they meet two specific conditions:

They share the same endpoint.

They extend in the exact same direction.

If these two conditions are satisfied, the rays are indistinguishable and are considered equal. For example, the rays AB → and AC → are different unless the points B and C lie along the same straight path originating from A.

Illustrative Analogy:

Imagine two runners starting in a relay race. Both start off from a common starting block and travel along an identical track - their paths being identical. Two rays may also be considered "directional twins," sharing an origin (endpoint) but pointing in opposite directions - making them geometrically indistinguishable from each other.

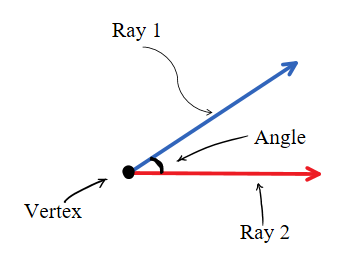
Rays and Angles

Formation of Angles by Rays

When two rays share a common endpoint, an angle is formed. The endpoint becomes the vertex of the angle, while the two rays extending outward are called the sides of the angle. For example, if two rays, OA → and OB →, share the endpoint O, they form the angle ∠AOB.

Angles can take various forms depending on the orientation of the rays:

Acute Angle: The rays form an angle smaller than 90°.

Right Angle: The rays form a perfect 90° angle.

Obtuse Angle: The rays form an angle greater than 90° but less than 180°.

Rays in Polygons

A polygon's sides can also be visualized as fragments of rays. Each side originates from one vertex and extends toward the next vertex, resembling segments of a ray that are limited in length.

A polygon can be seen as "stitched fragments of rays," where each ray has been synchronized to terminate at a specific endpoint—forming enclosed shapes like triangles or pentagons.

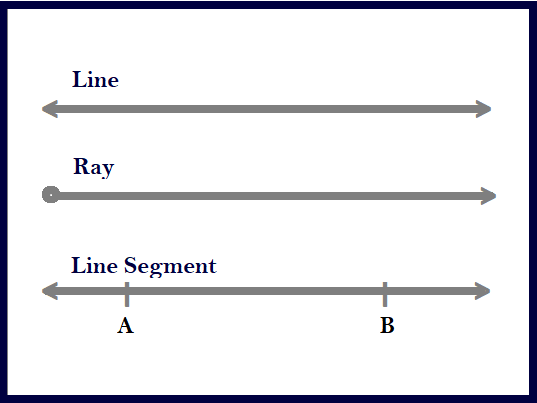
Comparing Rays, Lines, and Line Segments

Definition of Each

To comprehend the differences between lines, line segments, and rays, it's essential to start with their precise definitions:

Line

A line is an unending geometric form with no fixed start or finish points that continues on indefinitely from both directions without ever coming to an endpoint. A line's endless nature is often represented using two arrows on either end to symbolize its infinite nature.

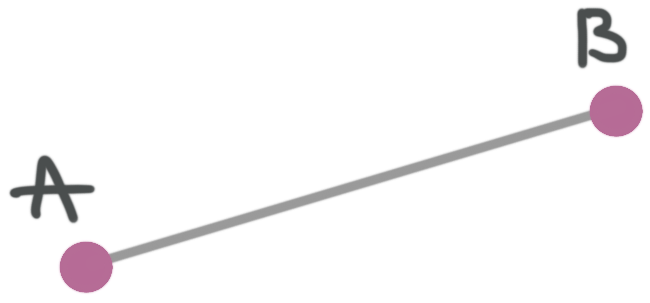
Line Segment 

Unlike a line, a line segment has two fixed endpoints and is finite in length. It represents a specific portion of a line with measurable distance between the two endpoints. Line segments are identified by the two points that bound them, for example, AB, where A and B are the endpoints.

Ray

A ray is a combination of the two, sharing properties of both lines and line segments. It has one fixed starting point, known as the endpoint, and extends infinitely in only one direction. Rays are denoted with a single arrow pointing in the direction of infinite extension, such as AB →, where A is the fixed starting point, and the ray travels infinitely toward B.

Visual Differentiation

To clearly differentiate between a line, a line segment, and a ray, visual representation is invaluable:

Line

A line is typically represented by a straight line with arrows on both ends, denoting its extension in both directions. For example, AB ↔ showcases that the line extends infinitely beyond A and B.

Line Segment

A line segment is drawn with two fixed points, often denoted as endpoints, and does not include arrows. Its representation, such as AB , indicates the finite portion of a line that exists between the points A and B.

Ray

A ray is represented with a single endpoint and an arrow at the other end to signify its direction of infinite extension. For example, AB → begins at point A (the fixed endpoint) and goes infinitely toward point B.

To better understand these distinctions, imagine all three originating from the same point:

The line extends infinitely to the left and right.

The line segment exists only between two set points on the line.

The ray starts at one point and extends infinitely in just one direction.

This kind of diagram is an excellent tool for visually comparing the three geometric forms.

Everyday Applications Comparison

Each of these geometric elements has analogies in real life, particularly when compared to different types of light sources:

Lines

Imagine lines as endless light paths extending in both directions - much like an abstract "beam of light" without an endpoint or source point - like an infinite beam illuminating both time axes from past to future, extending infinitely through infinity and back to the present. Timelines do indeed look much like infinite light paths!

Line Segments

The beam has a specific beginning and end, just as a line segment has two definite endpoints. The measurable length of the beam corresponds to the finite portion of the line it represents.

Rays

Rays are analogous to a car's headlights. When turned on, the light originates at a fixed point (the bulb of the headlight) and extends infinitely in one direction, illuminating the road ahead. The beam does not extend backward toward the car and thus perfectly resembles the geometry of a ray.

An analogy using light sources is especially helpful in understanding how geometric figures function: lines symbolize infinite extension in all dimensions; line segments have finite length and measurability, while rays only illuminate in one direction from their origin point, by creating such an engaging metaphoric framework for understanding abstract concepts more readily.

Role of Rays in Graphing Inequalities

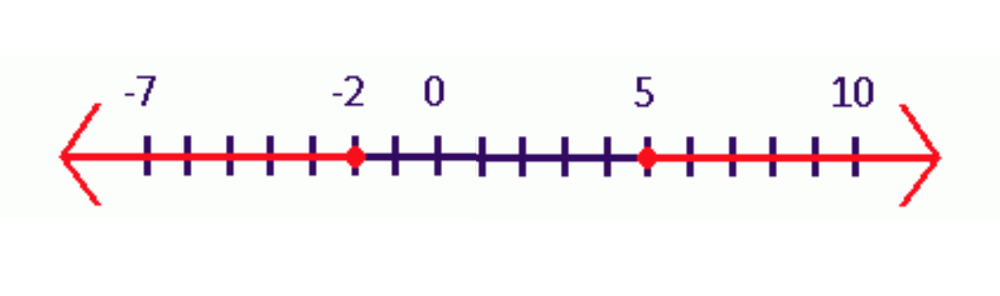
Rays on a Number Line

Rays play a crucial role in visually representing inequalities on a number line. For example, the inequality x ≥ 7 implies that x includes all values greater than or equal to 7, extending infinitely in the positive direction. On a number line, this inequality is represented by starting at the fixed point 7, marking it with a filled circle (to indicate that 7 is included), and drawing a ray extending to the right with an arrow to signify infinite extension.

Example : To represent x ≥ 7 :

Draw a number line.

Locate point 7 on the line and mark it with a filled circle.

Draw a ray starting at 7, pointing to the right with an arrow to show all values greater than 7.

Similarly, for inequalities like x > 7 , the representation changes slightly. Instead of a filled circle at 7, an open circle is used to indicate that 7 is not included, but the ray still extends infinitely to the right.

Exploring Its Significance

Rays on a number line provide an effective visual aid for understanding inequalities with unbounded growth or decreased potential. Their infinite extension emphasizes unbounded potential or progression - essential when understanding inequalities across multiple contexts.

Unique Application: Ray Geometry in Computer Graphics

Principles of Ray Tracing

Ray tracing in computer graphics relies on a central concept: the ray. Rays emanating from light sources interact with virtual objects to simulate light interactions; their reflection, refraction, and shadow creation result in hyper-realistic imagery.

Practical Uses in Gaming and Movies

Ray tracing has become the cornerstone of modern computer graphics, powering visual effects in movies like Avatar and photo realistic rendering in games like Cyberpunk 2077. By simulating real-world lighting through rays, these industries create immersive visual experiences.

Rays in graphics mimic our understanding of physical light but extend it infinitely in computational dimensions, connecting geometry with innovation.

Tips for Identifying Rays in Geometry Problems

Step-by-Step Approach

Observe the figure and look for a single endpoint connected to a line.

Check whether the line has an arrowhead on one end, signifying infinite extension.

Ensure the directionality is clearly marked by the arrow to distinguish it from other geometric elements.

Common Pitfalls to Avoid

Introduced the "Arrow Confusion Rule", students often misread an arrowhead as an endpoint instead of extension indicator, remember that each ray only needs one fixed endpoint and stick with this approach!

Conclusion

As one of the foundational concepts in geometry, rays provide unparalleled insights into mathematical elements. From their definition and practical applications, rays play an integral part in understanding angles, polygons, inequalities as well as computer graphics navigation among many other fields.

Rays represent more than static lines; they represent infinite possibilities in geometry, technological innovation, and everyday life. By starting small with just one point, we can imagine an inexhaustible potential of mathematical creativity that we may never exhaust in life itself.

Reference:

https://www.sciencedirect.com/journal/optics-and-lasers-in-engineering

https://developer.nvidia.com/discover/ray-tracing

https://link.springer.com/chapter/10.1007/978-3-540-75388-9_9